Hello! There are a few ways to find the derivative. One famous formula is below:
Note, t = x = 8
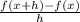
To find the derivative of s(t) = -9 - 3t let insert it into our formula:
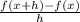
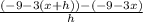
Insert x = 8

Do the Math

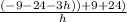
Group Like Terms
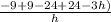
Cancel Terms and Finish Doing The Math
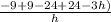
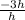
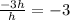
---------------------------------------------------------------------------------------
So when t = 8 the instantaneous velocity = -3