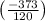In the first parenthesis, multiply the numerators so we get -12 and after multiply the denominator we will get 10
So the fraction in the first parenthesis will be
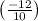
To solve the 2nd parenethesis, find the LCD of the denominator.
So the LCD of 5 and 3 = 15,
Now make the each denominator same as that of LCD.
So the new fractions in the 2nd parenthesis =
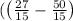 =
=

In the third parenthesis, multiply the numerators so we get 3 and after multiply the denominator we will get 8
So third parenthesis =
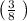
=
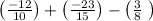
LCD of 12,15 and 8 is 120
=
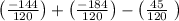
=