Question

HELP PLEAE

1) Divide.
4 ÷ 4/9

A) 9
B) 4
C) -9
D) 19


2) Give the range of the relation.

--------------
| X | Y |
| 4 | 3 |
| 8 | 6 |
| 12 | 9 |
| 16 | 12 |
--------------

A) {4, 12}
B) {4, 3}
C) {4, 8, 12, 16}
D) {3, 6, 9, 12}


4) Solve for g.
2g/5 + 4 = 8
Type your answer as an integer, like this: 17

A) g = -30
B) g = -10
C) g = 30
D) g = 10


5) The value of y varies directly with x, and y = 21 when x = 7. Find y when x = 4.

Type your answer as an integer, like this: 58.


6) Determine whether the ratios are proportional.
10/12 , 15/18

A) yes
B) No

7) Select which two transformations occur from the graph f(x) to the graph g(x). Be sure to select two answers.

f(x) = -3x and g(x) = -x + 1

A) translate 1 unit down
B) rotate about (0, 0) ; less steep
C) translate 1 unit up
D) rotate about (0, 0) ; steeper


8) Choose the correct solution for k.

k+7/5 > 6

A) k < 37
B) K > 37
C) k < 23
D) k > 23

9) Divide. -5/6 divide 1 2/3

Answer 1

the answer is a for #1

Answer 2

Hello!

Question 1: Divide 4 ÷
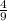`(4)/(9)`. Dividing a fraction is the same as multiplying its reciprocal.

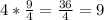`4 * (9)/(4) = (36)/(4) = 9`. The answer is A, 9.

Question 2: Find the range of the relation.

The range is the set of y-values. So therefore, we can list the y-values. From the given table: 3, 6, 9 and 12 are the y-values.

So therefore, the answer is D, {3, 6, 9, 12}.

Question 4: Solve for g. 2g/5 + 4 = 8

2g / 5 + 4 = 8 (subtract -4 from both sides)

2g / 5 = 4 (multiply both sides by 5)

2g = 20 (divide both sides by 2)

g = 10

Therefore, the answer is D, g = 10.

Question 5: The value of y varies directly with x, and y = 21 when x = 7. Find y when x = 4.

This statement says that y = kx, and in this case, we need to find k.

21 = k · 7 (divide both sides by 7)

k = 3 | Since we have k, we can substitute the value of k into the equation, y = kx.

y = 3x | Thus, we can find the value of y.

y = 3(4) → y = 12

Therefore, when x = 4, y = 12.

Question 6: Determine whether the ratios are proportional. 10/12 and 15/18

Proportions are an equation stating that two proportions are equal. So, we need to prove that 10/12 and 15/18 are proportionate to each other by simplifying the two ratios into simpler terms.

10/12 → 5/6 | 15/18 → 5/6

Since the ratios are equal, they are proportions of each other.

Question 7: Select which two transformations occur from the graph f(x) to the graph g(x). Be sure to select two answers.

f(x) = -3x and g(x) = -x + 1

Since a > 1, the graph of f(x) has a vertical stretch by a factor of 3, causing it to be steeper. While the graph of g(x) has no vertical stretch, causing it to be less steeper than f(x). Also, the graph of f(x) and g(x) is reflected. But, the graph of g(x) is translated 1 unit up.

So therefore, the answer is B, rotate about (0, 0) ; less steep, and C, translate 1 unit up.

Question 8: Choose the correct solution for k. When, (k + 7) / 5 > 6.

(k + 7) / 5 > 6 (multiply both sides by 5)

k + 7 > 30 (subtract both sides by 7)

k > 23

Hence, the answer is D, k > 23.

Question 9. -5/6 ÷ 1 2/3

To make the process more simple, we can convert 1 2/3, a mixed fraction, into 5/3, an improper fraction. As before, dividing by fractions is like multiplying its reciprocal.

-5/6 ÷ 5/3

-5/6 · 3/5

-15 / 30

-1/2 or -0.5

Therefore, the answer is -1/2 or -0.5.