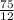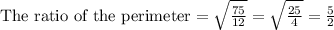Question

Two similar triangles have have areas of 75 m2 and 12 m2. find the ratio of the perimeters

Answer 1

we know that having similar triangles so the ratio of sides,area and volume are same.

`s/s=s^2/s^2`

we know that
`s^2` will be the area of triangle.

`s^2/s^2=75/12`

so
`s/s=√(75/12)`

so the ratio of the perimeter of two triangles will be

`(s+s+s)/(s+s+s)=(√(75) +√(75) +√(75) )/(√(12)+√(12) +√(12) )`

ratio of the perimeter=
`√(225) /√(36)`

Answer 2

The ratio of their perimeter is 5 : 2.

Explanation:

Since, if two triangles are similar,

Then, the ratio of their area is equal to the square of the ratio of corresponding sides or the ratio of the corresponding perimeters,

`\text{The ratio of their areas}=(\text{The ratio of the perimeter})^2`

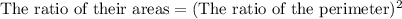
Here, the triangles have have areas of 75 m² and 12 m²,

So, the ratio of the area =
`(75)/(12)`

`(\text{The ratio of the perimeter})^2=(75)/(12)`

`\text{The ratio of the perimeter}=\sqrt{(75)/(12)}=\sqrt{(25)/(4)}=(5)/(2)`