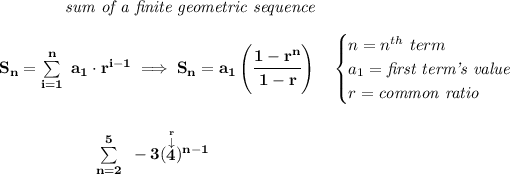
now, as you can see there, the common ratio is 4.
is it divergent or convergent?
well, the tale-tell fellow is the common ratio, if the common ratio is a fraction, is convergent, in this case it isn't, is 4, so is divergent.
a geometric serie is convergent only if the common ratio is a fraction, namely
0 < | r | < 1, is a value between 0 and 1.