Question

The slope of AB¯¯¯¯¯ is −4.

Which segments are parallel to AB¯¯¯¯¯ ?

Select each correct answer.

Answer 1

For two parallel lines slopes are equal.

Let us find slopes of each segment .

`slope=(y2-y1)/(x2-x1)`

(1) JK

`slope = (3-2)/(12-8)`

slope of JK =1/4

so JK is not parallel to AB

(2) CD

`slope=(10-2)/(4-6)`

slope=-4

slope of CD=slope of AD

so CD is parallel to AB

(3) EF

`slope =(5-9)/(2-1) = -4`

slope of EF=slope of AB

so EF is parallel to AB

(4) GH

`slope = (4-0)/(1-1)`

slope is undefined here

Answer 2

CD and EF

Explanation:

The slope of AB = -4

Two lines are said to be parallel if they have same slopes.

Slope =
`(y_2-y_1)/(x_2-x_1)`

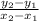
Option 1)J= (8,2) and K (12,3)

`(x_1,y_1)=(8,2)`

`(x_2,y_2)=(12,3)`

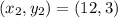
Slope =
`(y_2-y_1)/(x_2-x_1)`

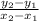
Substitute the values

Slope =
`(3-2)/(12-8)`

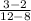
Slope =
`(1)/(4)`

Since the Slope of AB and JK is not equal . So, AB and JK are not parallel.

Option 2)C= (6,2) and D (4,10)

`(x_1,y_1)=(6,2)`

`(x_2,y_2)=(4,10)`

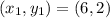
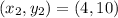
Slope =
`(y_2-y_1)/(x_2-x_1)`

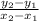
Substitute the values

Slope =
`(10-2)/(4-6)`

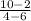
Slope =
`(8)/(-2)`

Slope =
`-4`

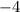
Since the Slope of AB and CD is equal. So, CD is parallel to AB.

Option 3)E= (1,9) and F (2,5)

`(x_1,y_1)=(1,9)`

`(x_2,y_2)=(2,5)`

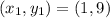
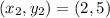
Slope =
`(y_2-y_1)/(x_2-x_1)`

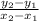
Substitute the values

Slope =
`(5-9)/(2-1)`

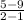
Slope =
`(-4)/(1)`

Slope =
`-4`

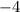
Since the Slope of EF and CD is equal. So, EF is parallel to AB.

Option 4)G= (1,0) and H (1,4)

`(x_1,y_1)=(1,0)`

`(x_2,y_2)=(1,4)`

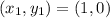
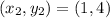
Slope =
`(y_2-y_1)/(x_2-x_1)`

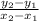
Substitute the values

Slope =
`(4-0)/(1-1)`

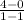
Slope =
`(4)/(1)`

Slope =
`4`

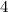
Since the Slope of GH and CD is not equal. So, GH is not parallel to AB.

The slope of AB is equal to the slopes of CD and EF

Hence CD and EF are parallel to AB