Question

Kurt has a jar of marbles. Six of the marbles are blue. The probability of randomly drawing a blue marble is 1/5. When Kurt adds more blue marbles to the jar, the probability of drawing a blue marble becomes 1/4. How man blue marbles did Kurt add to the jar? HELP PLZZZZ!!!!

A) 1
B) 2
C) 3
D) 4

Answer 1

Remark

Interesting question. One out every 5 marbles is blue. If you have 6 marbles in there then the fraction is 6/x. Your first step is to solve for x. Then you dilute the contents of the jar by adding a new number (x) of marbles and the probability becomes 1/4.

Step One

Find the number of marbles before any more were added.

1/5 = 6/x Cross multiply

x = 30 So there were 30 marbles to start with.

Step Two

Now he adds x marbles to both the blue and the total and the probability becomes 1/4. Set that up

`(6 + x)/(30 + x)=(1)/(4) \text{ Cross Multiply}`

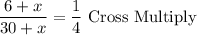
4(x + 6) = x + 30 Remove the brackets.

4x + 24 = x + 30 Subtract 24 from both sides.

4x = x + 30 - 24

4x = x + 6 Subtract x from both sides.

4x - x = 6

3x = 6 Divide by 3

x = 6/3

x = 2

Answer

He added 2 blue marbles. Note that you don't have to know anything about the rest of the marbles, except that they are not blue.