It is given that number of accidents on a particular highway is average 4.4 per year.
a. Let X be the number of accidents on a particular highway.
X follows Poisson distribution with mean μ =4.4
The probability function of X , Poisson distribution is given by;
P(X=k) =
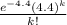
b. Probability that there are exactly four accidents next year, X=4
P(X=4) =
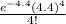
P(X=4) = 0.1917
Probability that there are exactly four accidents next year is 0.1917
c. Probability that there are more that three accidents next year is
P(X > 3) = 1 - P(X ≤ 3)
= 1 - [ P(X=3) + P(X=2) + P(X=1) + P(X=0)]
P(X=3) =

P(X=3) = 0.1743
P(X=2) =
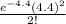
P(X=2) = 0.1188
P(X=1) =
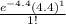
P(X=1) = 0.054
P(X=0) =
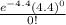
= 0.0122
Using these probabilities into above equation
P(X > 3) = 1 - P(X ≤ 3) = 1 - [ P(X=3) + P(X=2) + P(X=1) + P(X=0)]
= 1 - (0.1743 + 0.1188 + 0.054 + 0.0122)
P(X > 3) = 1 - 0.3593
P(X > 3) = 0.6407
Probability that there are more than three accidents next year is 0.6407