Answer:
it takes 2.4 seconds for the football to hit the ground
Explanation:
h = −16t^2 + 36t + 4
When the ball reaches the ground the height becomes 0
so we replace h with 0
0 = −16t^2 + 36t + 4
Apply quadratic formula to solve for t
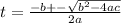
a= -16, b= 36 and c= 4
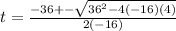
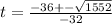
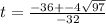
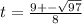
t= -0.106 and t= 2.356
time t cannot be negative
so it takes 2.4 seconds for the football to hit the ground