Question

David estimated he had about 20 fish in his pond. A year later, there were about 1.5 times as many fish. The year after that, the number of fish increased by a factor of 1.5 again. The number of fish is modeled by f(x) = 20(1.5)x.

Answer 1

After 2 years you have 45 fishes!
20x1.5 =30 after 1st year
30x1.5=45 after 2nd year

Answer 2

x = 3.97 years

Explanation:

I think your question is missed of key information, allow me to add in and hope it will fit the original one.

After how many years, the number of fish is 100.

My answer:

Given that, the equation that model this situation is

`f (x) = 20 (1.5) ^ x` where x is the number of years elapsed f(x) represents the amount of fish, 20 is the intinital value. 1.5 is the base number.

In how many years, the number of fish is 100? We need to set the equation equals 100 to find x:

`f (x) = 20 (1.5) ^ x = 100`

`100 = 20 (1.5) ^ x\\\\(100)/(20) = (1.5)^x\\\\ 5= (1.5)^x\\\\log_(1.5)(5) = log_(1.5)(1.5)^x\\\\log_(1.5)(5) = x\\\\x =log_(1.5)(5)\\\\x=3.97\ years`

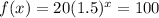
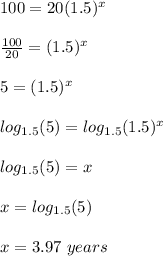
Hope it will find you well.