Please find the attached diagram for a better understanding of the question.
As we can see from the diagram,
RQ = 21 feet = height of the hill
PQ = 57 feet = Distance between you and the base of the hill
SR= h=height of the statue
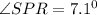 =Angle subtended by the statue to where you are standing.
=Angle subtended by the statue to where you are standing.
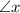 =
=
 which is unknown.
which is unknown.
Let us begin solving now. The first step is to find the angle
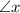 which can be found by using the following trigonometric ratio in
which can be found by using the following trigonometric ratio in
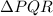 :
:
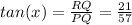
Which gives x to be:
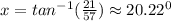
Now, we know that
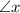 and
and
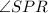 will get added to give us the complete angle
will get added to give us the complete angle
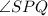 in the right triangle
in the right triangle
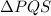 .
.
We can again use the tan trigonometric ratio in
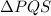 to solve for the height of the statue, h.
to solve for the height of the statue, h.
This can be done as:
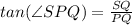
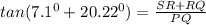
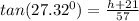
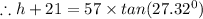
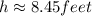
Thus, the height of the statue is approximately, 8.45 feet.