So remember that vertex form is
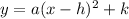
Firstly, put x^2 - 6x into parentheses:
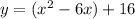
Next, to make what's inside the parentheses a perfect square, we need to divide the x coefficient by 2 and square that result. In this case: -6/2 = -3; (-3)^2 = 9. Add 9 into the parentheses, and subtract 9 on the outside of the parentheses:
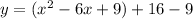
Next, factor (x^2-6x+9) to (x - 3)^2 and combine like terms outside of the parentheses, and your answer should be:
