keeping in mind that the vertex is "p" distance from either the focus or directrix, that means is half-way between both, check the picture below.
from -1 to -1/2 the distance is just 1/2 and half of that is 1/4.
now, if we go down from the directrix which is at -1/2, by 1/4 of a unit, we'll end up at
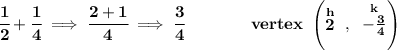
the distance "p" is obviously 1/4, however, since the parabola is opening downwards, is negative, therefore -1/4.

![\bf \begin{cases}h=2\\k=-(3)/(4)\\\\p=-(1)/(4)\end{cases}\implies 4\left( -(1)/(4) \right)\left[y- \left( -(3)/(4) \right) \right]=(x-2)^2\\\\\\-1\left( y+(3)/(4) \right)=(x-2)^2\implies y+\cfrac{3}{4} =-(x-2)^2\\\\\\y=-(x-2)^2-\cfrac{3}{4}](https://img.qammunity.org/2019/formulas/mathematics/middle-school/1wu0zuehs4mwpycggbd70eiguwp5pvjkb3.png)