Question

A horizontal spring on a frictionless surface has a spring constant of 10 N/m with a mass of 2kg attached to the end of the spring. If the spring is stretched 2m passed its point of equilibrium and released, how many times does the mass pass through equilibrium per second

Answer 1

0.356 times the mass pass through equilibrium per second.

Step-by-step explanation:

Given that,

Spring constant = 10 N/m

Mass = 2 kg

Stretched spring = 2m

We need to calculate the frequency

Using formula of frequency

`f=(1)/(2pi)\sqrt{(k)/(m)}`

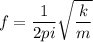
Where, m = mass

k = spring constant

Put the value into the formula

`f=(1)/(2\pi)\sqrt{(10)/(2)}`

`f=0.356\ Hz`

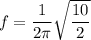
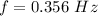
We know that,

Hertz = cycle per second

Hence, 0.356 times the mass pass through equilibrium per second.