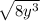*This is on the assumption that the equation is
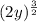
So firstly, you'll need to rewrite this as
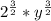
Next, remember the rule for exponents to radicals: "
![x^(m)/(n)=\sqrt[n]{x^m}](https://img.qammunity.org/2019/formulas/mathematics/middle-school/6822r1v0mjux6kutee250ny4ubof5fvgci.png) . Applying this rule to both would look like this:
. Applying this rule to both would look like this:
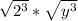
Next, simplify 2^3:
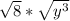
Next, apply the product rule of radicals (√ab = √a*√b) to this expression, and your answer will be