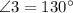Question

A pipe cleaner lay across a wire shelf. The wires that make up the shelf are parallel, and the pipe cleaner is a transversal. The parallel wires are labeled a, b, and, c, and the angles are labeled with numbers.The measure of one angle is 130°. Which statement is true regarding the 130° angle and angle 3?

A. They are same-side interior angles, so angle 3 measures 50°.
B. They are alternate interior angles, so angle 3 also measures 130°.
C. They are corresponding angles, so angle 3 also measures 130°.
D. They are alternate exterior angles, so angle 3 measures 50°.

Answer 1

The answer is B.

When a transversal cuts two parallel lines, there are 3 kinds of angle pairs which are congruent. Corresponding angles, alternate interior angles, and vertical angles.

In this case angle C is an alternate interior angle to 130°.

Answer 2

Answer: B. They are alternate interior angles, so angle 3 also measures 130°.

Explanation:

Here, according to the given figure, lines a and c are parallel lines.

And pipe cleaner is a transversal which is passing through to these parallel lines.

Therefore, by the property of parallel lines, the corresponding and alternative exterior or interior angles made by the common transversal ( pipe cleaner) on the lines a and c must be equal.

And, Here
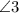`\angle 3` and
`130^(\circ)` are alternative interior angles by lines a and c with the same transversal, So, they must be equal.

Thus,
`\angle 3=130^(\circ)`