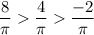Question

Please help !

Which function has the greatest rate of change on the interval from x = 0 to x = pi over 2?
f(x)
g(x)
h(x)
All three functions have the same rate of change.

Answer 1

The second option has the greatest rate of change

Answer 2

The function that has the greatest rate of change is:

g(x)

Explanation:

We know that the rate of change from x=a to x=b is determined as:

`(f(b)-f(a))/(b-a)`

We are asked to find the rate of change of each of the functions form x=0 to x=pi over 2.

f(x):

We are given that:

`f((\pi)/(2))=2`

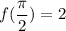
and,

`f(0)=0`

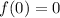
Hence,

`rate\ of\ change=(2-0)/((\pi)/(2)-0)\\\\\\rate\ of\ change=(4)/(\pi)`

g(x):

We have:

`g(0)=0`

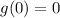
and

`g((\pi)/(2))=4`

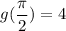
Hence,

`rate\ of\ change=(4-0)/((\pi)/(2)-0)\\\\\\rate\ of\ change=(8)/(\pi)`

h(x):

`h(x)=\sin (x-\pi)+5`

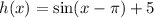
Now we have:

`h(0)=\sin (-\pi)+5\\\\h(0)=0+5\\\\h(0)=5`

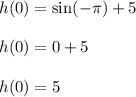
Also,

`h((\pi)/(2))=\sin ((\pi)/(2)-\pi)+5\\\\\\h((\pi)/(2))=\sin ((-\pi)/(2))+5\\\\h((\pi)/(2))=-\sin ((\pi)/(2))+5\\\\\\h((\pi)/(2))=-1+5\\\\h((\pi)/(2))=4`

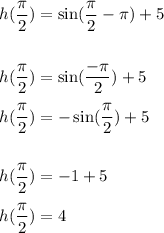
Hence, the rate of change is calculated as:

`rate\ of\ change=(4-5)/((\pi)/(2)-0)\\\\\\rate\ of\ change=(-2)/(\pi)`

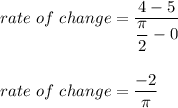
Hence, the greatest rate of change is:

g(x)

Since,

`(8)/(\pi)>(4)/(\pi)>(-2)/(\pi)`