Question

HURRYYYY !!!!! The graph of f(x) = 2x is shown on the grid. The graph of g(x) = ()x is the graph of f(x) = 2x reflected over the y-axis. Which graph represents g(x)?

Answer 1

Reflecting through x=0 maps x to -x so

g(x) =f(-x) = -2x

Answer: y = -2x

Can't see the choices, look for a downward slope of two units down for every unit to the right, through the origin.

Answer 2

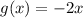`g(x)=-2x` and its graph is given below.

Explanation:

We are given that the function being transformed is
`f(x)=2x`.

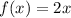Now, the new function g(x) is obtained by reflecting f(x) over the y-axis.

Since, 'Reflection over y-axis changes the function f(x) to f(-x)'.

So, we get,

`g(x)=f(-x)`

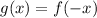
i.e.
`g(x)=2(-x)`

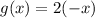
i.e.
`g(x)=-2x`

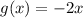
Hence, the graph of g(x) is given below.