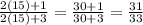Question

The numerator and the denominator of a certain fraction are consecutive odd numbers. If nine is subtracted frm the numerator, the ratio of the numerator to the denominator of the new fraction is two to three. What is the original fraction?

Answer 1

The denominator of a fraction is 1 more than 3 times the numerator. If the denominator is doubled and the numerator is increased by 2, the value of the resulting fraction is 1/4. Find the original fraction.

Answer 2

Any odd number can be written as the sum of an even number and 1, and every even number is an integer multiple of 2, so we can write the first odd number in our problem as
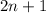`2n+1`, where n is some integer, and noting that consecutive odd numbers are 2 apart from one another, we can write the second odd number as
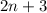`2n+3`. Our fraction then looks like

`(2n+1)/(2n+3)`

We're then told that subtracting 9 from the numerator makes the ratio 2-to-3, or mathematically:

`(2n+1-9)/(2n+3)=(2n-8)/(2n+3) =(2)/(3)`

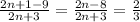
From here, we can cross-multiply to get the equation

`3(2n-8)=2(2n+3)`

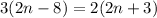
Which we can then solve for n:

`image`

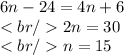
Substituting 15 in for our n in our original fraction, we find that

`(2(15)+1)/(2(15)+3) =(30+1)/(30+3) =(31)/(33)`