Question

Write an exponential equation for the following scenario, $1000 is deposited into an account that earns 5% interest.

A) y=1000(5)
B) y=1000(.05)^2
C) y=1000(1.05)^x
D) y=5(1000)^x

Answer 1

The formula to find the final amount A, compounded yearly for x year at r% when p is the initial amount :

`A=p(1+r)^x`

Given that, $1000 is deposited into an account that earns 5% interest.

So, p = 1000 and r =5% = 5/100= 0.05.

Next step is to plug in these values in the above equation to get the equation.

Hence,

`A = 1000(1+0.05)^x`

=
`1000(1.05)^x`

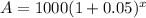
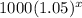
So, the correct choice is C) y=1000(1.05)^x.

Hope this helps you!