Question

NEEEED HELP!! A company gives each new salesperson a commission of $300 for the sale of a new car. The salesperson will receive a $100 increase for each addition car the person sells that week, so the person gets $400 for the next sale that week. Which equation can find the number of cars a salesperson must sell to earn $4,200 in a week? Recall that a1/d(n-1) is equivalent to an

Answer 1

B

Explanation:

Ed2021

Answer 2

General Idea:

In an arithmetic sequence, to find the
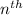`n^(th)` term, we need to use the below formula:

`a_n=a_1+(n-1)*d`

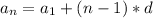
Here
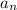`a_n` gives the
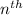`n^(th)` term

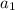`a_1` gives the first term of sequence

d is the common difference and n is the number of terms in the sequence.

Applying the concept:

In our problem it is given that "A company gives each new salesperson a commission of $300 for the sale of a new car",

so
`a_1=300`

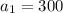
"The salesperson will receive a $100 increase for each addition car the person sells that week"

so
`d=100`

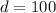
"find the number of cars a salesperson must sell to earn $4,200 in a week", this means that we need to find the value of n, when
`a_n=4200`

Setting up the equation based on the arithmetic sequence formula, we get:

`4200=300+(n-1)*100\\ 4200=300+100n-100\\ 4200=200+100n\\ 100n+200=4200\\ 100n+200-200=4200-200\\ 100n=4000\\ (100n)/(100)=(4000)/(100) \\ n=40`

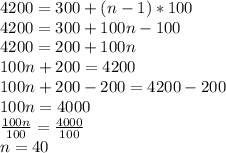
Conclusion:

New Salesperson has to sell 40 cars to earn $4200 in a week.