Question

What values for θ (0≤θ≤2π) θ ( 0 ≤ θ ≤ 2 π ) satisfy the equation: cos(θ) − tan(θ)cos(θ) = 0

Answer 1

When solving this problem any trigonometric equation, putting things in terms of sines and cosines is a good first step - mainly since most identities are built off these two functions.

`cos \theta - ((sin\theta)/(cos\theta) * cos\theta) = 0`

The cosines after the minus sign divide out leaving us with
`cos \theta - sin \theta = 0`.

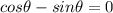If we subtract the sine to the other side, we are then asking a question: when are the cosine and the sine of angle equal? One common occurence is the 45 - 45 - 90 triangle when the cosine and sine of each angle is the same. So at 45 degrees, or
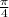`(\pi)/(4)` radians that happens. Saying this only tells half the story.

We now need to find when the sine and cosine have the same sign (since two negatives would work). Let's work quadrant by quadrant.

Quadrant I - sine and cosine are positive.

Quadrant II - sine is positive, cosine is negative

Quadrant III - cosine is negative, sine is negative

Quadrant IV - cosine is positive, sine is negative.

The 45-45-90 triangle is the Quadrant I response of 45 degrees. The quadrant III angle would be at 180 degrees plus that or 225 degrees. Since the problem is in radians, it would be [tex] \frac{\pi}{4} [/tex] and
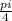`(\5pi)/(4)` since we add π radians to get around the unit circle.