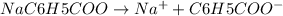
Here the base is a benzoate ion, which is a weak base and reacts with water.
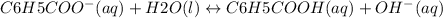
The equation indicates that for every mole of OH- that is produced , there is one mole of C6H5COOH produced.
Therefore [OH-] = [C6H5COOH]
In the question value of PH is given and by using pH we can calculate pOH and then using pOH we can calculate [OH-]
pOH = 14 - pH
pH given = 9.04
pOH = 14-9.04 = 4.96
pOH = -log[OH-] or
![[OH^(-)] = 10^{^(-pOH)}](https://img.qammunity.org/2019/formulas/chemistry/high-school/bjgs60v16w8ycpd3rd0p0carhy1zo9xa1q.png)
![[OH^(-)] = 10^{^(-4.96)}](https://img.qammunity.org/2019/formulas/chemistry/high-school/nhiwdlp461g4bwt6d3y6xv1ldrx3wsioi4.png)
![[OH^(-)] = 1.1* 10^(-5)](https://img.qammunity.org/2019/formulas/chemistry/high-school/wleevrtgu28l0wejeyv4d5thtfwhodlegi.png)
The base dissociation equation kb =
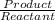
![kb =([C6H5COOH][OH^(-)])/([C6H5COO^(-)])](https://img.qammunity.org/2019/formulas/chemistry/high-school/gpihculccs9vnr7bozhaferbtl2l9nmk2j.png)
H2O(l) is not included in the 'kb' equation because 'solid' and 'liquid' are taken as unity that is 1.
Value of Kb is given =
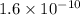
And value of [OH-] we have calculated as
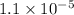 and value of C6H5COOH is equal to OH-
and value of C6H5COOH is equal to OH-
Now putting the values in the 'kb' equation we can find the concentration of C6H5COO-
![kb =([C6H5COOH][OH^(-)])/([C6H5COO^(-)])](https://img.qammunity.org/2019/formulas/chemistry/high-school/gpihculccs9vnr7bozhaferbtl2l9nmk2j.png)
![1.6* 10^(-10) = ([1.1* 10^(-5)][1.1* 10^(-5)])/([C6H5COO^(-)])](https://img.qammunity.org/2019/formulas/chemistry/high-school/15mrjt15py8565b6kgbujhxdael8nufiud.png)
![[C6H5COO^(-)] = ([1.1* 10^(-5)][1.1* 10^(-5)])/(1.6* 10^(-10))](https://img.qammunity.org/2019/formulas/chemistry/high-school/lslvujztvkd5njjub254rx3xdrq2kw8fkc.png)
![[C6H5COO^(-)] = 0.76 M](https://img.qammunity.org/2019/formulas/chemistry/high-school/al95jrqix7suvmokachegrndjr2rav48x3.png) or
or
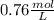
So, Concentration of NaC6H5COO would also be 0.76 M and volume is given to us 0.50 L , now moles can we calculated as : Moles = M X L
Moles of NaC6H5COO would be =
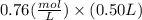
Moles of NaC6H5COO (sodium benzoate) = 0.38 mol