Answer:
The correct option is C.
Explanation:
The given inequality is
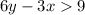
Isolate y on the left side in the above inequality.
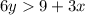
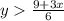
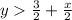
If an inequality is defined as
 , then the shaded region is above the solid line.
, then the shaded region is above the solid line.
If an inequality is defined as
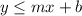 , then the shaded region is below the solid line.
, then the shaded region is below the solid line.
If an inequality is defined as
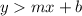 , then the shaded region is above the dashed line.
, then the shaded region is above the dashed line.
If an inequality is defined as
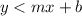 , then the shaded region is below the dashed line.
, then the shaded region is below the dashed line.
Since the sign of inequality is >, therefore the shaded region is above the dashed line.
Hence option C is correct.