This is a Bayes Theorem Problem
P(oil | negative test) P(negative test)= P(negative test | oil) P(oil)
P(oil | negative test) = P(negative test | oil) P(oil) / P(negative test)
P(oil | negative test) =P(negative test | oil) P(oil) / ( P(negative test | oil) P(oil) + P(negative test | no oil) P(no oil) )
We're given the prior probability of oil, P(oil)=.45, so P(no oil)=.55
We given P(negative test | no oil) = 0.80, so P(negative test | oil) = .20
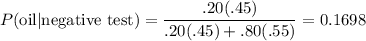
Choice A