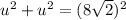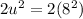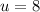This is the isosceles right triangle, the diagonal of a square, the thing that so upset the Pythagoreans. The two sides and diagonal of a square are in ratio
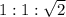 so we get
so we get
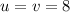
We could have also gotten this using Trig:
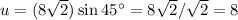
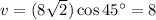
Or by recognizing u=v because remaining angle is 45 so this must be isosceles so