Question

What is the probability of flipping a coin 8 times and getting heads 3 times?

A.21.9%
B.10.9%
C.3.1%
D.27.3%

Answer 1

I'm pretty sure the answer is D. 27.3%

because say you still flipped it 8 times but got heads 4 times, theres a 50% chance of that happening because there are two sides to the coin, you can pretty much just eyeball it from there.

Answer 2

The probability of flipping a coin 8 times and getting heads 3 times is 21.9%

Explanation:

Given

A coin

A coin has two sides (a head and a tail)

The probability of getting a head is equal to the probability of getting a tail;

Let these probabilities be represented by H and P;

i.e. H = Probability of getting a Head

T = Probability of getting a Tail

Since both probabilities are equal and probability always sum to 1 then

H + T = 1

H + H = 1

2H = 1

H = 0.5 and T = 0.5

P (3 heads in 8 tosses) is given by the binomial representation

`\left[\begin{array}{c}&n\\&r\end{array}\right] * H^(r) * T^(n - r)`

Where n = number of tosses = 8

r = number of heads

By Substitution,

`\left[\begin{array}{c}&n\\&r\end{array}\right] * H^(r) * T^(n - r)` becomes

`\left[\begin{array}{c}&8\\&3\end{array}\right] * 0.5^(3) * 0.5^(8 - 3)`

`(8!)/((8-3)!3!) * 0.5^(3) * 0.5^(8 - 3)`

`(8 * 7 * 6 * 5!)/((5!!3!) * 0.5^(3) * 0.5^5`

`(8 * 7 * 6 )/(3 * 2) * 0.5^(3) * 0.5^5`

`8 * 7 * 0.5^(3) * 0.5^5`

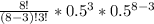
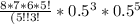
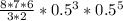
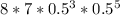
= 0,21875

= 21.875%

= 21.9% --- Approximately