Answer: The present value of the given annuity is $ 11040.13.
Step-by-step explanation:
To calculate the present value for a given annuity, we use the equation:
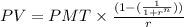
where,
PV = present value
PMT = fixed annuity amount = $1500
r = rate of interest = 6 % = 0.06
n = time period = 10 years
Putting values in above equation, we get:
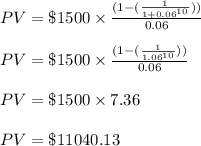
Hence, the present value of the given annuity is $ 11040.13.