Question

A spherical object has a density ρ. if it is compressed under high pressure to quarter of its original diameter, its density will now be

Answer 1

The density will increase by a factor of 64:
`\rho_2=64\rho_1`

Step-by-step explanation:

Hello,

Density at the first state is defined as ratio between mass and volume:

`\rho_1 =(m)/(V_1)`

The volume of a sphere at the initial condition is:

`V_1=(4)/(3) \pi  r_1^3`

If the diameter is quartered we compute volume after the compression as:

`V_2=(4)/(3) \pi (r_2)^3\\r_2=(D_2)/(2)=((D_1)/(4) )/(2)  =(D_1)/(8) =(2r_1)/(8)=(r_1)/(4)\\V_2=(4)/(3) \pi ((r_1)/(4))^3\\V_2=(4)/(3) \pi(r_1^3)/(64)\\V_2=(1)/(48) \pi r_1^3`

In such a way, the volume is reduced therefore the density is increased by a factor of:

`(V_2)/(V_1)= ((1)/(48) \pi r_1^3)/((4)/(3) \pi  r_1^3) =(1)/(64)\\ V_2=(1)/(64)V_1`

Finally, as the volume is decreased by a factor of 1/64, the density is increased by a factor of 64 by considering:

`\rho_2 =(m)/(V_2)=(m)/((1)/(64)V_1) =64(m)/(V_1)\\ \rho_2=64\rho_1`

Best regards.

Answer 2

Answer: its new density would be 0.016 times that of its original.

Explanation:

The density of a spherical object can be calculated using following formula

ρ = 4/3 x
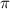`\pi` x r³

Here r is the radius of the spherical object

and
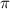`\pi` is a mathematical constant having value 22/7

Let's assume the original diameter of the object is d.

Therefore its radius will be = d/2 = 0.5d

Let us plug in this value and find density ρ₁

ρ₁ = 4/3 x
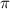`\pi` x (r)³

ρ₁ = 4/3 x 22/7 x (0.5d)³

Now we are compressing the object so that its diameter becomes one quarter of the original.

The new diameter of the object would be d/4 = 0.25d

Therefore the new radius would be 0.25d/2 = 0.125d

Let us find the new density ρ₂

ρ₂ = 4/3 x 22/7 x (0.125d)³

Let us compare ρ₁ and ρ₂ now

ρ₂/ρ₁ = ( 4/3 x 22/7 x (0.125d)³) / ( 4/3 x 22/7 x (0.5d)³ )

We can cancel out 4/3 and 22/7 here

ρ₂/ρ₁ = (0.125d)³ / (0.5d)³

d gets cancelled.

ρ₂/ρ₁ = 0.01562

ρ₂ = 0.01562 ρ₁

ρ₂ is new density and ρ₁ is original density

Therefore we can say that its new density would be 0.016 times that of its original.