Answer:
1) 25/8 or 3 1/8 M&Ms 2) No, it won't last forever 3)
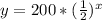 4) The quantity of chocolate decreases exponentially at a rate of 1/2, for a each period of time.
4) The quantity of chocolate decreases exponentially at a rate of 1/2, for a each period of time.
Explanation:
This problem works like a Geometric Sequence, whose quotient is 1/2.
Supposing that Isabella has eaten 1/2 and has bee each day eating only half of what's been left.
Setting on a Table:
Day | Candy
1 | 200
2 | 100
3 | 50
4 | 25
5 | 12.5
6 | 6.25
7 | 3.125
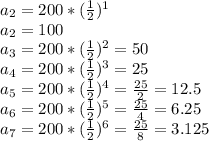
2) The equation used is the Recursive Formula for the Geometric Sequence, adjusted to the data:
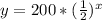
3) Check the graph below
4) The quantity of chocolate decreases exponentially at a rate of 1/2, for a each period of time. Each day the amount goes getting smaller and smaller till it gets to zero. Then you can insert more values in the Domain you'll get zero, this is the reason why the curve goes closer and closer until gently intercepts x-axis.