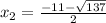Question

Which are the solutions of x^2 = –11x + 4?

Answer 1

This is a quadratic equation, i.e. an equation involving a polynomial of degree 2. To solve them, you must rearrange them first, so that all terms are on the same side, so we get


`x^2 + 11x - 4 = 0`

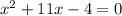
i.e. now we're looking for the roots of the polynomial. To find them, we can use the following formula:


`x_(1,2) = (-b\pm√(b^2-4ac))/(2)`

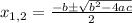
where
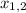`x_(1,2)` is a compact way to indicate both solutions
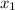`x_1` and
`x_2`, while
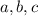`a,b,c` are the coefficients of the quadratic equation, i.e. we consider the polynomial
`ax^2+bx+c`.

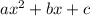So, in your case, we have
`a=1,\ \ b=11,\ \ c=-4`

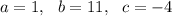
Plug those values into the formula to get


`x_(1,2) = (-11\pm√(121+16))/(2) = (-11\pm√(137))/(2)`

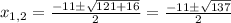
So, the two solutions are


`x_1 = (-11+√(137))/(2)`


`x_2 = (-11-√(137))/(2)`