Answer:
Option D. 9.2 units.
Explanation:
In the ΔJKL ⇒ ∠J + ∠K + ∠L = 180
∠J + 67 + 74 = 180° ⇒ ∠J = 39°
In the given triangle JKL we apply law of sines
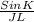 =
=
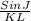 =
=

Now we take
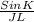 =
=
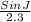
 =
=
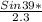
JL =
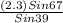
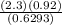 = 3.36 units
= 3.36 units
Now
 =
=
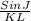
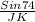 =
=
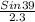
JK =
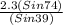
=
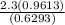
= 3.51 units
So perimeter of the triangle = 3.36 + 3.51 + 2.3 = 9.15 units ≈ 9.2 units.
Option D is the answer.