Question

The table shows the percentage of users of a data tablet who returned the product to an Internet provider within a year after purchase, after a year, and the reasons for the return. Find the probability of a user returning the tablet within a year, given that a new version of the tablet was introduced.

Battery life Monthly service fee New version Totals
Within a year 5% 25% 60% 90%
After a year 1% 3% 6% 10%
Totals 6% 28% 66% 100%

Answer 1

. 90

Explanation:

__

. 90

Answer 2

The data has been properly arranged in a Two-Way Table and attached in the image below.

We are to find the probability of a user returning the tablet within a year, given that a new version of the tablet was introduced. Let A be the event that user returns the tablet within a year and B be the event that the new version of tablet was introduced. So we have to find the probability of A given B. Mathematically this can be written as:

P(A|B)

The formula for P(A|B) is:

`P(A|B)=(P(A\&B))/(P(B))`

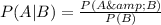
P(A&B) means probability of both A and B occurring at the same time. In context of this problem P(A&B) means probability that user returned the tablet within a year because and new version was introduced. This probability is marked red in the image below and is equal to 60%.

P(B) means probability of occurrence of event B. In context of this problem P(B) means the probability that new version was introduced. This probability has been marked green in the image below and is equal to 66%.

Using the values in the above formula, we get:

`P(A|B)=(60\%)/(66\%)=0.909=90.9\%`

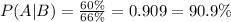
Thus, the probability of a user returning the tablet within a year, given that a new version of the tablet was introduced is 90.9%