Question

I’m confused on this one

Answer 1

In a parallelogram, the opposite angles will be congruent to each other.

In this parallelogram, angles B and C are congruent, and angles A and D are congruent.

We will work on angles B and C first. Set the two angles to equal each other:

`12x + 6 = 6x + 66`

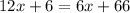
Subtract 6 from both sides:

`12x = 6x + 60`

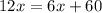
Subtract 6x from both sides:

`6x = 60`

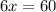
Divide both sides by 6 to get x by itself:

`\boxed{x = 10}`

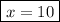
x will equal 10.

Set angles A and D to equal each other:

`54 = 3y`

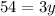
Divide both sides by 3 to get y by itself:

`\boxed{y = 18}`

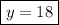
y will equal 18.

The x and y values that make this quadrilateral a parallelogram will be x = 10, and y = 18.