So remember that all exterior angles add up to 360, so what you are gonna do is add all the exterior angles together and equal it to 360, and it'll look like this:

Firstly, combine like terms. Your equation will look like this:

Next, subtract 126 on each side. It'll look like this:
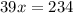
Finally, divide 39 on each side, and then your answer should be
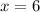
To find the measure of C, just substitute 6 in x, then solve.

In short, the measure for Vertex C's exterior angle is 50 degrees.