Question

Question part points submissions used this exercise involves the formula for the area of a circular sector. find the area of a sector with central angle 3π/5 rad in a circle of radius 9 m. (round your answer to one decimal places.)

Answer 1

The formula for the area of a sector in radians is
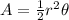
`A= (1)/(2)r^2 \theta`, where theta is the angle in radians. For us, the formula looks like this:
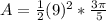
`A= (1)/(2)(9)^2* (3 \pi )/(5)`. Doing all the multiplication on that gives us
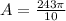
`A= (243 \pi )/(10)`. Multiplying in pi and rounding to the nearest tenth gives us an area of 76.3 meters squared. You can use the formula for the area of a sector with the angle in degrees as well. Just replace the 360 degrees with 2pi and it works the exact same way.