Answer:
63.565 inch³
Explanation:
A candle manufacturer sells cylindrical candles in sets of three.
Each candle in the set is of different size.
Smallest candle has a radius of
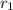 = 0.5 inches and a height of
= 0.5 inches and a height of
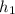 = 3 inches.
= 3 inches.
other two candles are with scale factors of 2 and 3.
It means radius and height for second candle are
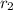 = 0.5 × 2 = 1 inch and
= 0.5 × 2 = 1 inch and
 = 3 × 2 = 6 inches.
= 3 × 2 = 6 inches.
Radius and height of third candle are
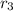 = 0.5 × 3 = 1.5 inch and
= 0.5 × 3 = 1.5 inch and
 = 2 × 3 = 6 inches
= 2 × 3 = 6 inches
Now we have to calculate the amount of wax needed to create one set of candles.
Volume of Candle 1 =
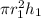
=
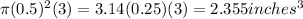
Volume of Candle 2 =
![\pi r_(2)^(2)h_(2)^[2]](https://img.qammunity.org/2019/formulas/mathematics/middle-school/iioi5uz0hg5t25q2jjc283z0zc419pbulv.png)
=
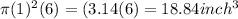
Volume of Candle 3 =
![\pi r_(3)^(2)h_(3)^[2]](https://img.qammunity.org/2019/formulas/mathematics/middle-school/ewjoaahxfxiyuj7rd2smq087r2smyfoyx9.png)
=
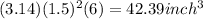
Total quantity of wax = Volume (1) + volume (2) + volume (3)
= 2.355 + 18.84 + 42.39 = 63.565 inch³
63.565 inch³ wax is needed to create one set of candles.