Question

[IMPORTANT PLEASE READ!!] I am currently at home, I'm in summer school for Algebra 1. I'm taking a pre-test, once I finish this pre-test successfully I can leave summer school. Please help.

Instructions: Select the correct answer from the drop-down menu.

Consider the absolute value function: f(x)=-|x+2|-2

The vertex of the function is: (All the answers that are listed)

(2,-2)
(-2,2)
(-2,-2)
(2,2)

Answer 1

Answer: (-2,-2)

Explanation:

The parent function of the absolute function is f(x)=|x|.

General form of absolute function :
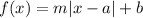`f(x)=m|x-a|+b` , where (a,b) is the vertex of the function.

Also , when m<0 then the graph opens downwards.

when m>0 then the graph opens upwards.

Given : The absolute value function:
`f(x)=-|x+2|-2`

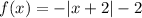
Comparing the given absolute function to with the general form of the absolute function , then we get

m= -1<0 , a= -2 and b= -2

Since m is negative , it means the graph of the function opens downwards .

∴, the vertex of the function is : (-2,-2)

Answer 2

The vertex of the function is:

(-2,-2)

Explanation:

We are given a absolute value function f(x) in terms of variable "x" as:

`f(x)=-|x+2|-2`

We know that for any absolute function of the general form:

`f(x)=a|x-h|+k`

the vertex of the function is : (h,k)

and if a<0 the graph of function opens downwards.

and if a>0 the graph of the function opens upwards.

Hence, here after comparing the equation with general form of the equation we see that:

a= -1<0 , h= -2 and k= -2

Since a is negative , hence, the graph opens down .

Hence, the vertex of the function is:

(-2,-2)