Question

The area of the following equilateral triangle is 62.4 square feet that is he height

Answer 1

The height of this triangle would be 10.4

In order to find this, you first must find the length of the sides. Using a manipulated formula for area of an equilateral triangle, we can determine the lengths of the side. Below if the formula.

S =
`(2)/(3)3^{(3)/(4)} √(A)`

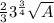
In this, S is the length of the side and A is the area. So we plug in and get:

S =
`(2)/(3)3^{(3)/(4)} √(62.4)`

S =
`(2)/(3)3^{(3)/(4)} 7.89`

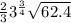
S = 12

Now that we have the side as 12, we can use the Pythagorean Theorem to find the height. If you split a equilateral triangle down the middle, you are left with two right triangles. Using this right triangle, the hypotenuse would be 12, the first leg would be 6 (half of the base) and the height would be the other leg. So we plug in and solve.

`a^(2) + b^(2) = c^(2)`

`6^(2) + h^(2) = 12^(2)`

`36 + h^(2) = 144`

`h^(2) = 108`

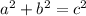
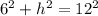
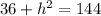
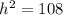
h = 10.4