Question

Which of the follow function is the graphed below?

Answer 1

The equation is a piecewise function written as such.

f(x) =
`\left \{ {{x^2 + 6 , when x \ \textless \ 2} \atop {-x + 6, when x \geq 2}} \right.`

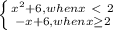
In order to find these, you need to find the graph of each individual portion of the graph as if they were separate. Start with the parabola.

Since this is a basic x^2 graph, we first must see if it has any shifts other than just moving up 6 places (because this is where the y-intercept is). So we start with the graph of x^2 + 6 and try other points to see if it satisfies the equation. We'll try 2.

x^2 + 6
(2)^2 + 6
4 + 6
10

And if you look on the graph, it hits the point (2, 10), so we know it must not be changed in any way other than moving up 6 spots. Then we look for the values of x in which to use this graph. Since there is an open dot on x = 2, we know not to include 2 and only to include what is less.

For the second part of the graph, we'll need to pick a couple points that allow us to find the value of the line. (3, 3) and (6, 0) are both on the graph. So we'll use those values to first find the slope of the new line.

m = (y1 - y2)/(x1 - x2)
m = (0 - 3)/(6 - 3)
m = -3 / 3
m = -1

Now that we have the slope, we can use that and a point to find the intercept.

y = mx + b
0 = -1(6) + b
0 = -6 + b
6 = b

So that gives us the equation y = -x + 6 and we know that the closed circle means to include x = 2 and everything after it.