Question

The discriminant of the function is...

Answer 1

The discriminant is a way of figuring out how many zeros a quadratic function has.

The function is graphed, so we can visually see how many zeros it has. There are two zeros in the function.

The discriminant will be less than 0 if there are two zeros.
The discriminant will equal 0 if there is one zero.
The discriminant will be greater than 0 if there are no zeros.

Since this function has two zeros, the discriminant will be negative.

Answer 2

Answer: the correct option is (B) Positive.

Step-by-step explanation: We are given to select the correct option about the discriminant of the graphed function.

We see that

the graph shows a quadratic function, since there are two ends and both goes on the same side.

We know that

for a function
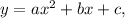`y=ax^2+bx+c,` the discriminant is given by

`D=b^2-4ac.`

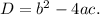
Also,

the quadratic equation will have

(i) one real root if D = 0,

(ii) two real roots if D > 0,

(iii) two unreal roots if D < 0.

From the graph, we see that there are two solutions of f(x) = 0. These are

x = -3 and x = -1.5.

Therefore, the given function will have two real roots. This implies that the discriminant of the graphed function is positive.

Option (B) is CORRECT.