Question

Jason hired a luxury car service. The service charges a fixed rate of $4.92, regardless of the number of miles traveled, plus an additional $3.79 per mile. If Jason wants to spend no more than $4.20 per mile, what is the minimum number of miles he should travel?

Answer 1

12 miles

12 miles on a car is like fresh from the factory

Answer 2

The minimum number of miles he should travel is 12 miles.

Explanation:

Suppose, the number of miles, Jason should travel is
`x`

The service charges a fixed rate of $4.92 and an additional $3.79 per mile.

So, the total charge for
`x` miles will be:
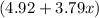`(4.92+3.79x)` dollars.

Jason wants to spend no more than $4.20 per mile. That means, for
`x` miles he wants to spend
`\$4.20x`

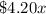
So, the inequality will be.....

`4.92+3.79x\leq 4.20x\\ \\ 4.92\leq 4.20x-3.79x\\ \\ 4.92\leq 0.41x\\ \\ 0.41x\geq 4.92\\ \\ x\geq (4.92)/(0.41) \\ \\ x\geq 12`

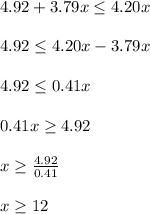
So, the minimum number of miles he should travel is 12 miles.