Question

Find the standard form of the equation of the parabola with a focus at (-2, 0) and a directrix at x = 2. (5 points) y2 = 4x 8y = x2 x = 1 divided by 8 y2 y = 1 divided by 8 x2

Answer 1

The standard form of the equation of the parabola is
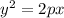
`y^2=2px`, where p is distance between parabola focus and directrix and parabola branches go in positive direction of x-axis.
Since focus is at (-2, 0) and a directrix at x = 2, the distance between parabola focus and directrix is 4 units. The parabola vertex lies at the origin and the branches go in negative direction of x-axis (because focus lies on the left side from directrix). Then the equation of parabola is:


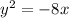`y^2=-8x`.