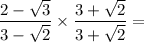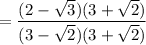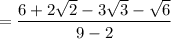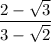
What you need to do is to rationalize the denominator.
You are not supposed to leave a root in the denominator,
so you need to change the form of this fraction so that there
is no root in the denominator.
The process to do this is calling "rationalizing the denominator."
You will end up with an integer in the denominator.