Answer: f(x) =x³ + 1 is not an odd function
Explanation:
We are asked about odd functions
If f(x) be a function and f(-x) =-f(x)
then f(x) is an odd function
1) f(x) = sinx
Here f(-x) = sin(-x)
=-sinx sin(-x) = -sinx
Therefore it is an odd function
2) f(x) = sin2x
f(-x) = sin 2(-x)
= sin (-2x)
=-sin2x = -f(x)
Therefore it is an odd function
3) f(x) = x³ +1
f(-x) = (-x)³ + 1
= -x³+1
For odd function it should be -(x³+1)
Hence it is not an odd function
4) f(x) =

f(-x) =

=
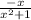
= -f(x)
Therefore it is an odd function
5) f(x) = ∛(2x)
f(-x) =∛(-2x)
= - ∛(2x)
=- f(x)
Hence it is an odd function
∴ 3) f(x) = x³+1 is not an odd function