To begin to solve this problem, we must first note that when you are doing n + (-z), it is equal to n - z. Now, knowing this, we need to begin to find the LCD of 8 and 2. The LCD of 8 and 2 is 8, so now we need to turn -

into something over 8. -

becomes -

. Now, we can subtract the numerators over a common denominator. -7 - 4 is -11, so -

+ (-

) is -
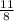
.