Question

Arrange these functions from the greatest to the least value based on the average rate of change in the specified interval.Tilesf(x) = x2 + 3xinterval: [-2, 3]f(x) = 3x - 8interval: [4, 5]f(x) = x2 - 2xinterval: [-3, 4]f(x) = x2 - 5interval: [-1, 1]Sequence>>>

Answer 1

By definition, the average rate of change is given by:

`AVR = (f(x2)-f(x1))/(x2-x1)`

We evaluate each of the functions in the given interval.

We have then:

For f (x) = x ^ 2 + 3x:

Evaluating for x = -2:

`image`

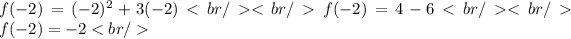
Evaluating for x = 3:

`image`

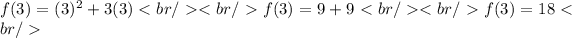
Then, the AVR is:

`AVR = (18-(-2))/(3-(-2))`

`AVR = (18+2)/(3+2)`

`AVR = (20)/(5)`

`AVR = 4`

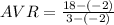
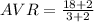
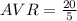
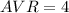
For f (x) = 3x - 8:

Evaluating for x =4:

`image`

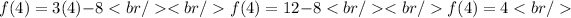
Evaluating for x = 5:

`image`

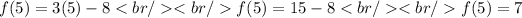
Then, the AVR is:

`AVR = (7-4)/(5-4)`

`AVR = (3)/(1)`

`AVR = 3`

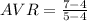
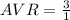
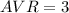
For f (x) = x ^ 2 - 2x:

Evaluating for x = -3:

`image`

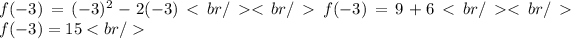
Evaluating for x = 4:

`image`

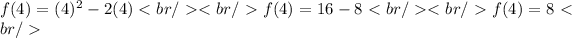
Then, the AVR is:

`AVR = (8-15)/(4-(-3))`

`AVR = (-7)/(4+3)`

`AVR = (-7)/(7)`

`AVR = -1`

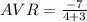
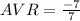
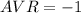
For f (x) = x ^ 2 - 5:

Evaluating for x = -1:

`image`

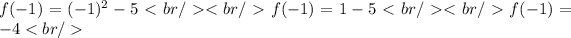
Evaluating for x = 1:

`image`

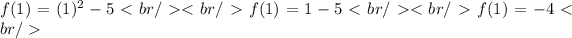
Then, the AVR is:

`AVR = (-4-(-4))/(1-(-1))`

`AVR = (-4+4)/(1+1)`

`AVR = (0)/(2)`

`AVR = 0`

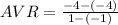
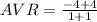
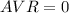
Answer:

from the greatest to the least value based on the average rate of change in the specified interval:

f(x) = x^2 + 3x interval: [-2, 3]

f(x) = 3x - 8 interval: [4, 5]

f(x) = x^2 - 5 interval: [-1, 1]

f(x) = x^2 - 2x interval: [-3, 4]