Question

I need help on a mathematics question. I need an explanation.

Solve 2 log x = log 64.

x = 8
x = ±8
x = 32
x = 128
Why is it not B and rather A? I agree with 8, but I also think that -8 works.

Answer 1

To solve this problem you must apply the proccedure shown below:

1- To solve the logarithm expression
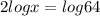`2 log x = log 64`, you must apply the following properties:

`alogx=logx^(a)`

`logx-logy=log(x/y)`

`a^(log(a)x)=x`

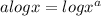
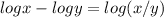
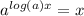
2-Keeping this on mind, you have:

`log x^(2)-log 64=0\\ log(x^(2) /64)=0\\ 10^{log(x^(2) /64)} =10^(0) \\ x^(2) /64=1`

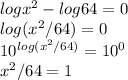
3. Now, solve for
`x`:

`x=√(64)\\ x=8, x=-8`

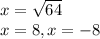
4. If you susbtitute
`x=-8` into the expression shown in the problem, you will see that it is invalid.

Therefore. the answer is:
`x=8`.