Answer:
CT = DT = 4 units.
Explanation:
The diagonals of a parallelogram bisect each other, this means that the point of intersection is the midpoint for each one of them.
So, diagonal CR has coordinates C(5,-2) and R(1,2). Its midpoint would be
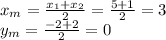
Therefore, the mid point is at (3,0).
Now, to find the length of CT and DT, we use the definition of distance between two points:
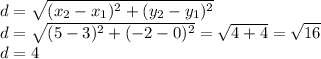
Therefore, the length of CT is 4 units. DT has the same length, because as we said, T divides diagonal is equal parts because is a mid point.
So, CT = DT = 4 units.