Question

Based on the information marked in the diagram, ABC and DEF must be congruent.

Answer 1

Hello!

As you can see, both AB and DE both have one dash mark. This means they are congruent. Angles C and F are congruent as well. A and D both have right angles. These triangles are congruent.

I hope this helps!

Answer 2

Option A is correct

True, ΔABC and ΔDEF must be congruent.

Step-by-step explanation:

LA theorem or Postulates states that given two right triangles, where one acute angle and a leg of one of the triangles are congruent to an angle and a leg of the other triangle, then the two triangles are congruent.

In ΔABC and ΔDEF

AB = DE [Leg] [Given in the figure]

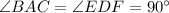`\angle BAC = \angle EDF = 90^(\circ)` [Given]

`\angle BCA = \angle EFD` [Acute angle] [Given in the figure]

Then, by the LA theorem or Postulates;

therefore, ΔABC
`\cong` ΔDEF .